VOLUME 6 NUMBER 1 (January to June 2013)
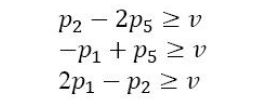
Philipp. Sci. Lett. 2013 6 (1) 016-020
available online: January 31, 2013
*Corresponding author
Email Address: gdavid@science.upd.edu.ph
Received: October 28, 2012
Revised: December 9, 2012
Accepted: December 10, 2012
ARTICLE
A Variation of Discrete Silverman'sGame with Varying Payoffs
by Guido David* and Richele S. De Asis
Institute of Mathematics, and
Computational Science Research Center
University of the Philippines
Diliman, Quezon City
Computational Science Research Center
University of the Philippines
Diliman, Quezon City
A discrete form of Silverman's game, a two-playerzero sum game, is played with each playerchoosing a number from 1 to n. Each player's goalis to choose the larger number as long as it is lessthan three times the opponent's chosen number.Here we consider a variation of Silverman's game, wherein thepayoff to the player choosing the larger number is the differencebetween the two numbers, but if the larger number is at leastthree times the smaller number, the payoff to the player choosingthe smaller number is twice the difference between the twonumbers. Analysis of payoff matrices and the Minimax Theoremare used to solve the game. In both versions of the game, resultsshow that when n = 3 the unique optimal strategy reduces eachplayer's choices to just three numbers. The difference betweenthe two solutions is in the probabilities each player must selectthe three choices.
© 2025 SciEnggJ
Philippine-American Academy of Science and Engineering